-
海缆损坏大多是由于渔业活动、航运活动、海洋工程作业等人为因素造成的。为降低海缆损坏的风险，通常认为最经济、最有效的海缆保护方式是进行埋设保护。近年来，随着海洋经济的发展，海上工程活动的增加[1-2]，国内外许多专家学者在海底电缆保护及埋深方面做了大量研究工作[3-5]。目前的方法大体上分成4类：（1）经验法；（2）概率法模型；（3）能量法力学模型；（4）试验及数值模拟法。其中，文献[6]基于经验要求：水下电缆不得悬空于水中，浅水区埋深不宜小于0.5 m，深水航道区埋深不宜小于2 m。文献[7]利用能量法利用简单的物理模型，理论分析锚的整个下落过程的运动状态，计算出锚的海底入土深度。文献[8]针对常用的大抓力锚，基于砂土和黏土中的室内模型试验，开展了不同土质条件中的下落深度研究。文献[9]基于太沙基极限承载力公式，运用能量法通过建立船锚落深的理论算法，并通过最小二乘法拟合试验数据，得出计算船锚刺入砂土深度。文献[10]基于模型试验和数值模拟的方法分析拖锚过程中船锚的啮土轨迹，提出船锚啮土深度与影响范围的理论计算方法。文献[11]描述锚泊作业的不同情况，基于概率分析，提出了锚与锚链撞击管道的概率及撞击能量的计算公式。文献[12-14]总结出各个国家和地区对海底管线挖埋深度的要求。但目前各行各业对海底电缆及管线的埋深都没有一个明确的方法，不同行业不同设计院对电缆及管线埋深设计的理解也不尽相同。
本文将在文献[7]基础上，基于能量法推导船锚垂直撞击海底入土深度的简化数学模型，并与实测数据对比探讨该数学模型在不同抗剪强度的海底基质、不同水深情况下的适用性及适用范围。
-
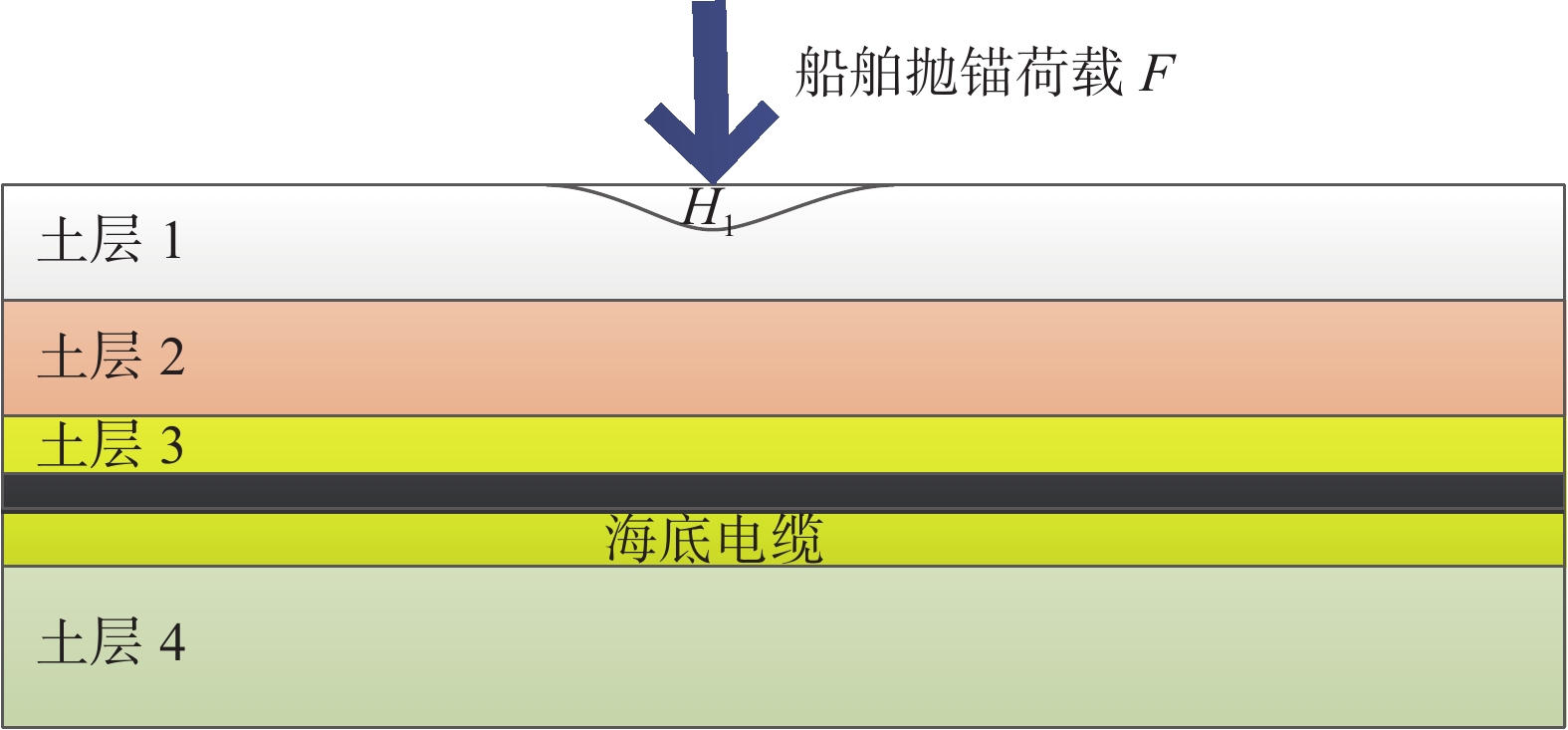
首先，在建立数学模型过程中，将船锚的抛锚、落锚的整个过程划分为以下3个阶段进行模拟[7]：（1）锚从初始位置到刚入水的瞬间；（2）锚从入水到锚落到海底表层；（3）锚从海底表层到最终贯入。以下将根据以上三个阶段的情况，建立相应模型。如图1所示。
图 1 锚下落运动示意图
Figure 1. Movement diagram of falling anchor
为了简化计算，上述数学模型进行了一些假设：（1）假设船锚的阻力全部来自海底土体剪切力，忽略了船锚入土时来自正面的端阻力；（2）假设船锚为自由落体，未考虑海水流速及船只控制对船锚下落速度的影响。
-
船锚在自由抛锚的情况下，不考虑使用锚机刹车情况下进行抛锚。根据能量守恒定理，可以得到锚刚入水的速率按式（1）计算，如果采用锚机刹车，则
$ {v}_{1} $ 根据锚机设定的速度考虑。$$ {v}_{1}=\sqrt{2{\rm{g}}{h}_{1}} $$ (1) 式中：
$ {h}_{1} $ ——锚在空气中下降到水面的高度（m）。 -
锚在水中受重力
$ m{\rm{g}} $ 、浮力$ {F}_{{\rm{B}}} $ 及流体阻力$ f $ 作用。锚在水线下的受力方程为：$$ m{\rm{g}}-{F}_{{\rm{B}}}-f=m\dfrac{{\rm{d}}v}{{\rm{d}}t} $$ (2) 根据锚的重量可以推算出锚的体积，并计算出相应的浮力大小。
假设将锚的下落近似成标准球体下落的过程，则锚近似为球体的等效半径为r，锚在水中的阻力可按式（3）进行计算：
$$ f=kv=6{\text{π}} \eta rv $$ (3) 式中：
$ \eta $ ——海水的粘度（Pa·s）；$r=\sqrt[3]{\dfrac{3V}{4{\text{π}} }}$ ——锚的近视计算等效半径（m）；$ v $ ——锚在海水中的速率（m/s）。将式（3）代入式（2）方程两边同时除以k，得：
$$ \dfrac{m{\rm{g}}-{F}_{{\rm{B}}}}{k}-v=\dfrac{m}{k}\dfrac{{\rm{d}}v}{{\rm{d}}t} $$ (4) 令
$K=\dfrac{m{\rm{g}}-{F}_{{\rm{B}}}}{k}$ ，代入上式积分后解得：$$ {v}_{2}=K-(K-{v}_{1}){{\rm{e}}}^{-\dfrac{k}{m}T} $$ (5) 由于
${\rm{d}}x=v{\rm{d}}t$ ，可得
${\displaystyle \int }_{0}^{h2}{\rm{d}}x={\displaystyle \int }_{0}^{T}[K-(K-{v}_{1}\left){{\rm{e}}}^{-\frac{k}{m}T}\right]{\rm{d}}t$ 。解得
$ {h}_{2} $ ：$$ {h_2} = TK - \left[ {\left( {K - {v_1}} \right){{\rm{e}}^{ - \dfrac{k}{m}T}}} \right]/\left( { - \dfrac{k}{m}} \right) + \left[ {\left( {K - {v_1}} \right)} \right]/\left( { - \dfrac{k}{m}} \right) $$ (6) 如果采用锚机刹车，则
$ {v}_{2} $ 计算值如果大于锚机设定的速度，则按锚机设定速率考虑。 -
锚从海底表面到最终的入土深度，考虑锚运动至海底表面的瞬间全部动能被锚所受到的阻力吸收。而不同的锚在不同的海底基质所受到的阻力不同，受力特性也不同，在此基础上进行简化，不考虑海底基质端阻力，将这部分作为安全储备，只考虑锚入土过程产生的剪切破坏，通过土体的剪切变形吸收船锚的动能，根据功能原理，冲击能等于土壤不排水抗剪强度吸收能力，由
$ \overline{F}={\int }_{0}^{h}\tau A{\rm{d}}h $ 代入能量公式可得式（7）入土深度：$$ h=\dfrac{m{v}_{2}^{2}}{2\overline{F}} $$ (7) 式中：
$ h $ ——锚垂直入海底土层的入土深度（m）。其中：对于粘性土
$\tau =c+\sigma \rm{tan}\varphi$ 或$\tau =\dfrac{{q}_{{\rm{u}}}}{2}$ 。 -
某一海域海深20 m，取平均水温20 ℃，落锚为570 kg的无杆锚（霍尔锚），以船锚从水面落下为基准，海底土壤抗剪强度假设为50 kPa。为了简化计算模型，将船锚简化为六面体，底面长宽同锚宽最大尺寸，高度同锚爪同高。本例中B=371 mm，L=950 mm，H=862 mm。
其中水的粘度
$ \eta $ 随温度变化而变化，其中在水温20 ℃时，$ \eta $ =1.002×10-3 Pa·s$$ V=\dfrac{m}{{\rho }_{\mathrm{锚}}}=\dfrac{570}{7\;850}=0.072\;6\;{{\rm{m}}}^{3} $$ $$ {F}_{{\rm{B}}}={\rho }_{\mathrm{水}}{V}_{\mathrm{锚}}\text{g=1\;000}\times \text{0.072\;6}\times \text{10=726\;N} $$ $$ r=\sqrt[3]{\dfrac{3V}{4{\text{π}}}}=\sqrt[3]{\dfrac{3\times 0.072\;6}{4{\text{π}} }}=0.26\;{\rm{m}} $$ $$ k=6{\text{π}} \eta r=6\times 3.14\times 1.002\times 1{0}^{-3}\times 0.25=4.91\times 1{0}^{-3}\;{\rm{Pa}}\cdot {\rm{s}}\cdot {\rm{m}} $$ $$ K=\dfrac{m{\rm{g}}-{F}_{{\rm{B}}}}{k}=\dfrac{570\times 10-726}{4.91\times 1{0}^{-3}}=1\;013\times 1{0}^{3}\;{\rm{m}}/{\rm{s}} $$ $$ {v}_{2}=K-(K-{v}_{1}){{\rm{e}}}^{-\dfrac{k}{m}T} =18.7\;{\rm{m}}/{\rm{s}} $$ 故入土深度为：
$$ h=\dfrac{m{v}_{2}^{2}}{2\overline{F}}=\dfrac{570\times 18.{7}^{2}}{2\times 50\;000\times (0.371+0.95)\times 2\times 0.862}=0.87\;{\rm{m}} $$ -
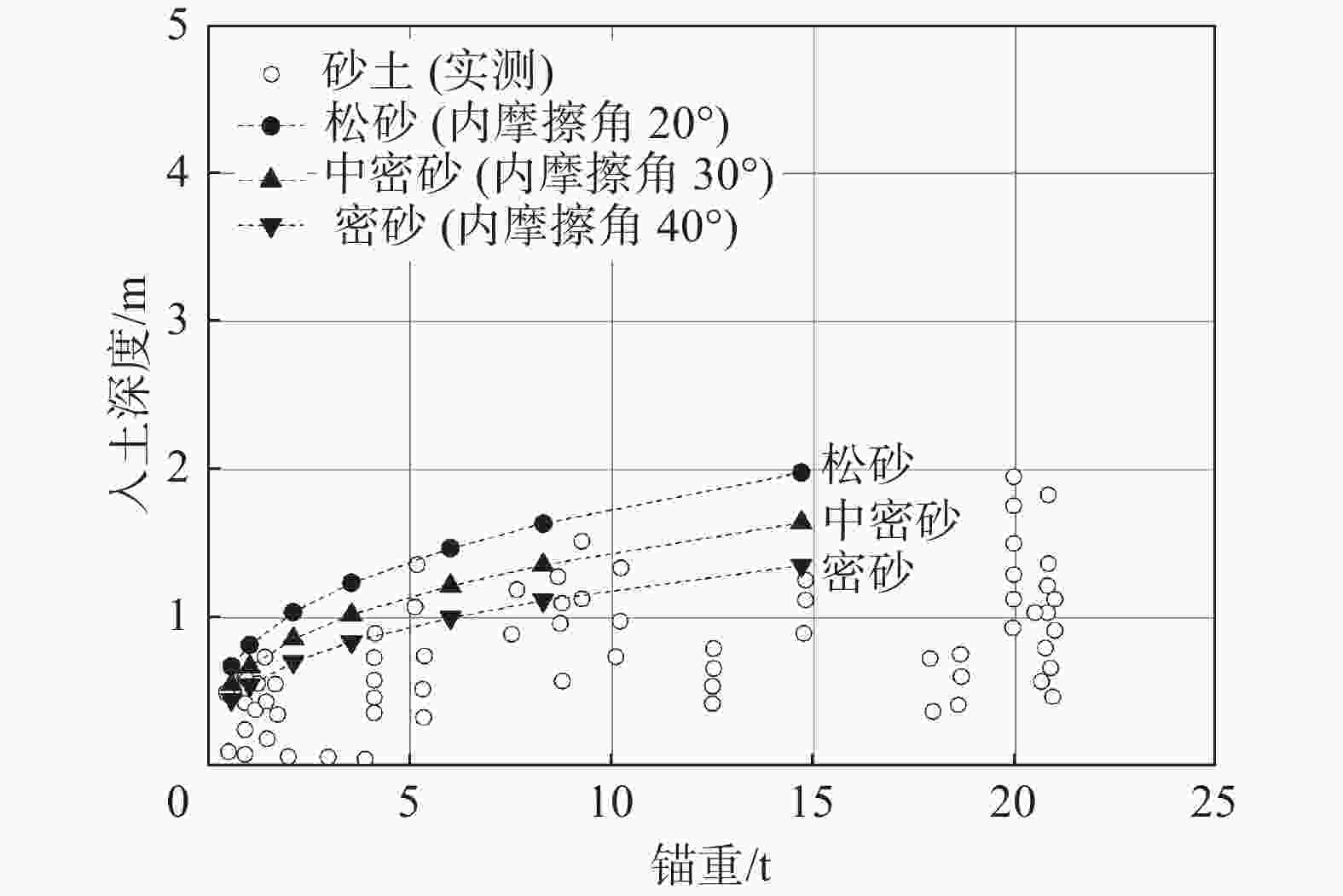
对锚重在570 kg~1.47 t的船锚，以20 m水深自由落锚，计算锚在海底土壤抗剪强度为20 kPa~100 kPa时的入土深度。同时将该计算结果与《城市电力电缆线路设计技术规定》（DL/T 5221－2016）条文说明[15]中引用的日本运输省港湾技术研究所的NO.215《投锚与入土深度试验》[16]现场实测报告值进行了对比。如图2、图3所示。
图 2 锚重与入土深度（粘土）
Figure 2. Anchor weight and depth of penetration in clay
图 3 锚重与入土深度（砂土）
Figure 3. Anchor weight and depth of penetration in sand
由图3可看出实测结果具有一致的规律性，在土体抗剪强度大的情况下，曲线斜率小，入土深度随锚重变化小；土体抗剪强度小的情况下，曲线斜率大，入土深度随锚重变化大。可以看出对于粘性土而言抗剪强度在20 kPa～100 kPa区间，计算值与实测基本较吻合。这主要是由于在粘性土中，土体的剪切变形由不排水抗剪强度控制，应力-应变曲线呈应变硬化型，船锚的贯入深度由锚重及土体剪切强度决定，抗剪强度越大，剪切变形越小。对于粘土抗剪强度在20 kPa～30 kPa之间时，由于入土深度变化幅度较大，且实测数据偏少，建议在工程应用时增加试验论证。
因此，该计算方法在海底土壤为粘性土时抗剪强度大于20 kPa，计算结果在实际工程中具有应用价值。
-
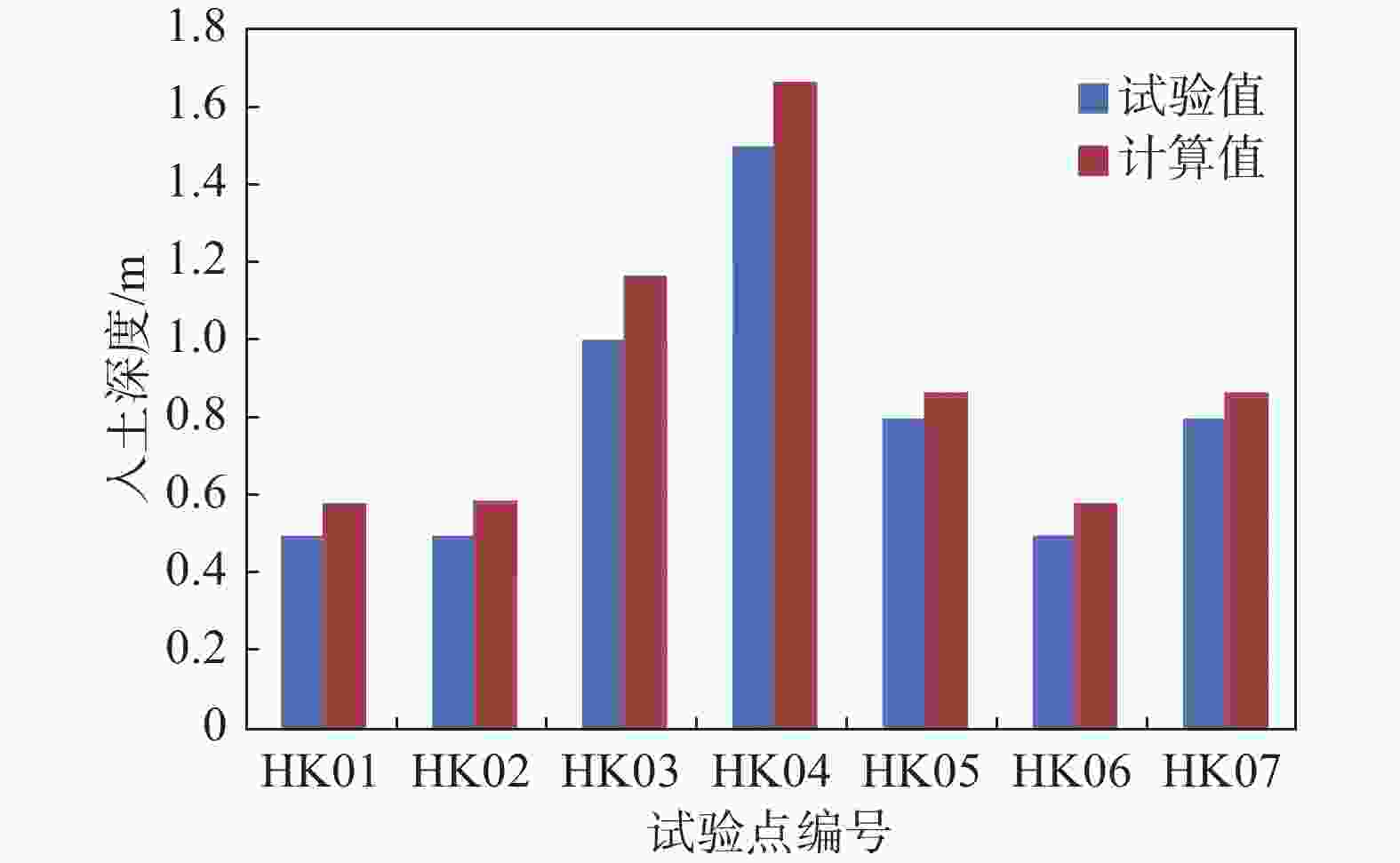
南澳863柔直示范项目海缆工程在进行路由勘探时，利用钻探船船锚对沿线7个点的船锚入土深度进行了现场试验，同时在相同条件下采用数学模型进行了理论计算，如表1所示。两者的对比情况如图4所示。
表 1 勘测期间钻探船抛锚船锚入土深度统计表
Table 1. Statistics of anchor depth of drilling ship during survey
勘探点号 HK1 HK2 HK3 HK4 HK5 HK6 HK7 水深/m 3.51 5.07 7.93 10.14 9.95 10.06 11.05 海底底质类型 粉砂/淤泥 粉砂/淤泥 淤泥/淤泥质土 淤泥/淤泥质土 粉砂/淤泥质土/粉土 粉砂/粉土 粉砂/粉土 土质抗剪强度/kPa 22 30 15 15 25 40 28 实测船锚入土深度/m 0.5 0.5 1.0 1.5 0.8 0.5 0.8 计算船锚入土深度/m 0.58 0.59 1.17 1.66 0.87 0.58 0.87 
图 4 试验值与计算值对比情况
Figure 4. Comparison of test values and calculated values
由表1及图4所示，采用数学模型计算的结果均高于现场实测值，偏差在8%～18%之间。这主要是由以下原因引起的：（1）数学模型进行了简化计算，假设船锚的阻力全部来自海底土体剪切力；（2）忽略了船锚入土时来自正面的端阻力，并将船锚简化为均匀的柱体考虑侧阻力。因此，计算结果与实测值比较会偏大。
-
通过上文的数学模型计算方法与实测结果比较分析可以得出以下结论：
1）该简化计算模型适用于抗剪强度大于20 kPa的粘性土计算船锚入土深度。
2）该计算模型忽略了端部阻力的影响，计算结果偏大8%~18%，在工程应用中，可将该部分作为设计裕度考虑，计算结果对敷设保护方案的影响在可接受范围。
3）影响船锚入土深度的边界条件是多方面的，包括船锚类型、重量、水深、抛锚方式、海底土质、流速等因素。为了能够满足特定条件下的计算需求，仅考虑部分主要边界条件得到的计算模型难免存在一定差异。
4）该方法还有待在实践中积累不同实测数据进行验证和拟合修正，并结合经验法和概率法进行分析，为海底电缆敷设保护提供设计依据。
Simplified Calculation Method and Applicability of Anchor Depth
-
摘要:
目的 随着海洋经济的发展，越来越多海上风电送出、海上油气平台的供电、岛屿联网供电项目涉及海底电缆的敷设。目前主要的敷设保护方式为直埋敷设，但不考虑经济性的埋深设计显然是不合理的。为了能有效地保护海底电缆，又能合理控制投资，需要在项目开发阶段就确定海缆的合理埋设深度。因此，在缺乏足够的设计输入资料的情况下，文章提出了一种简化的船锚入土深度计算方法，为项目前期确定敷设保护方案提供依据。 方法 将船锚入土过程分成三个阶段建立数学模型，并对船锚入土过程进行了一系列边界条件假设。在此基础上，基于能量法对各个阶段船锚运动过程进行力学模型分析和推导，提出了考虑海底基质剪切破坏的船锚入土深度简化计算方法。通过将该方法的计算值与不同情况下的实测值进行对比分析，探讨该计算方法的适用性及适用范围。 结果 通过该简化方法计算值与实测结果比较分析表明，该计算方法适用于抗剪强度大于20 kPa的粘土的情况下计算船锚入土深度，且该方法的计算误差在可接受范围内。 结论 从计算结果对比分析可以看出，该简化计算方法对工程实践应用具有指导意义，但该方法还有待在实践中积累不同实测数据进行验证和拟合修正，并结合经验法和概率法进行分析，为海底电缆敷设保护提供设计依据。 Abstract:Introduction With the development of Marine economy, more and more projects of offshore wind power transmission, offshore oil and gas platform power supply and island network power supply involve laying submarine cables. At present, the main laying protection method is direct burial laying, but the buried depth design without considering the economy is obviously unreasonable. In order to effectively protect the submarine cable and reasonably control the investment, it is necessary to determine the reasonable burial depth of the submarine cable in the project development stage. Therefore, in the absence of sufficient design input data, this paper proposes a simplified calculation method for the depth of anchor penetration, which provides a basis for determining the laying protection scheme in the early stage of the project. Method In this paper, the anchor penetration process was divided into three stages to establish mathematical models, and a series of boundary conditions were assumed for the anchor penetration process. On this basis, based on the energy method, we analyzed and deduced the mechanical model of the anchor movement process at each stage, and proposed a simplified calculation method of the anchor penetration depth considering the shear damage of the seabed substrate. By conducting a comparative analysis of the calculated values of the method and measured values under different situations, we explored the applicability and scope of the calculation method. Result The comparison between the calculated value of this simplified method and the measured result shows that this calculation method is suitable for calculating the depth of anchor penetration in the case of clay with shear strength greater than 20 kPa, and the calculation error of this method is within the acceptable range. Conclusion From the comparative analysis of the calculation results in this paper, it can be seen that the simplified calculation method has guiding significance for the application in engineering practice, but the method still needs to accumulate different measured data in practice for verification and fitting correction, and combine the empirical method and probabilistic method for further analyses, to provide design basis for submarine cable laying protection. -
Key words:
- submarine cable /
- laying protection /
- anchoring /
- energy method /
- shear strength
-
表 1 勘测期间钻探船抛锚船锚入土深度统计表
Tab. 1. Statistics of anchor depth of drilling ship during survey
勘探点号 HK1 HK2 HK3 HK4 HK5 HK6 HK7 水深/m 3.51 5.07 7.93 10.14 9.95 10.06 11.05 海底底质类型 粉砂/淤泥 粉砂/淤泥 淤泥/淤泥质土 淤泥/淤泥质土 粉砂/淤泥质土/粉土 粉砂/粉土 粉砂/粉土 土质抗剪强度/kPa 22 30 15 15 25 40 28 实测船锚入土深度/m 0.5 0.5 1.0 1.5 0.8 0.5 0.8 计算船锚入土深度/m 0.58 0.59 1.17 1.66 0.87 0.58 0.87 
下载: 导出CSV
-
[1] 常胜, 李炬添, 张振, 等. 海上风电场联合送出优化方案研究 [J]. 南方能源建设, 2019, 6(2): 49-53. DOI: 10.16516/j.gedi.issn2095-8676.2019.02.009. CHANG S, LI J T, ZHANG Z, et al. Research on offshore wind farm optimization scheme for joint delivery [J]. Southern Energy Construction, 2019, 6(2): 49-53. DOI: 10.16516/j.gedi.issn2095-8676.2019.02.009. [2] 崔东岭, 江春, 史忠秋, 等. 大型海上风电项目中的集电海缆研究 [J]. 南方能源建设, 2020, 7(2): 98-102. DOI: 10.16516/j.gedi.issn2095-8676.2020.02.015. CUI D L, JIANG C, SHI Z Q, et al. Research on array submarine cables in large offshore windfarm [J]. Southern Energy Construction, 2020, 7(2): 98-102. DOI: 10.16516/j.gedi.issn2095-8676.2020.02.015. [3] 王亚东, 伍林伟, 高彬, 等. 砂质海床条件下海底电缆埋深研究 [J]. 南方能源建设, 2020, 7(3): 81-88. DOI: 10.16516/j.gedi.issn2095-8676.2020.03.010. WANG Y D, WU L W, GAO B, et al. Research on the burial depth of submarine cable in sandy seabed [J]. Southern Energy Construction, 2020, 7(3): 81-88. DOI: 10.16516/j.gedi.issn2095-8676.2020.03.010. [4] 吴庆华, 马凌, 岳浩, 等. 500 kV海南联网工程海底电缆线路设计 [J]. 上海电气技术, 2021, 14(3): 5-9+43. WU Q H, MA L, YUE H, et al. Design of submarin cable line of 500 kV Hainan power link project [J]. Journal of Shanghai Electric Technology, 2021, 14(3): 5-9+43. [5] 张冲. 海上风电项目长距离海缆敷设ROV后冲埋施工探析 [J]. 福建建筑, 2021(9): 41-43. ZHANG C. Exploration and analysis of burying construction by ROV after laying for long-distance submarine cable in offshore wind power project [J]. Fujian Architecture & Construction, 2021(9): 41-43. [6] 中华人民共和国住房和城乡建设部. 电力工程电缆设计标准: GB 50217—2018 [S]. 北京: 中国计划出版社, 2018. Ministry of Housing and Urban-Rural Development of the People′s Republic of China. Standard for design of cables of electric power engineering: GB 50217−2018 [S]. Beijing: China Planning Press, 2018. [7] 庄元, 宋少桥. 海底管线埋深问题研究 [J]. 大连海事大学学报, 2013, 39(1): 61-64. DOI: 10.16411/j.cnki.issn1006-7736.2013.01.004. ZHUANG Y, SONG S Q. Study on the depth of submerged pipeline [J]. Journal of Dalian Maritime University, 2013, 39(1): 61-64. DOI: 10.16411/j.cnki.issn1006-7736.2013.01.004. [8] 陈峰, 汪嘉钰, 别亦白, 等. 不同土质条件下大抓力锚的落锚深度研究 [J]. 港工技术, 2017, 54(5): 43-48. DOI: 10.16403/j.cnki.ggjs20170512. CHEN F, WANG J Y, BIE Y B, et al. Study on penetration depth of high holding power anchors under different soil conditions [J]. Port Engineering Technology, 2017, 54(5): 43-48. DOI: 10.16403/j.cnki.ggjs20170512. [9] 杜颖, 陈峰, 徐伟. 砂土基质下落锚深度试验研究及计算方法 [J]. 南方能源建设, 2018, 5(增刊1): 222-227. DOI: 10.16516/j.gedi.issn2095-8676.2018.S1.040. DU Y, CHEN F, XU W. Experimental study and calculation method of falling anchor depth under sand soil [J]. Southern Energy Construction, 2018, 5(Supp. 1): 222-227. DOI: 10.16516/j.gedi.issn2095-8676.2018.S1.040. [10] 徐伟, 汪嘉钰, 郑志源, 等. 船舶拖锚对海底电缆埋深的影响 [J]. 船海工程, 2018, 47(1): 147-150+154. DOI: 10.3963/j.issn.1671-7953.2018.01.033. XU W, WANG J Y, ZHENG Z Y, et al. Influence of dragging anchor upon the buried depth of submarine cable [J]. Ship & Ocean Engineering, 2018, 47(1): 147-150+154. DOI: 10.3963/j.issn.1671-7953.2018.01.033. [11] 郭振邦, 张群站, 徐慧. 海底管道关于锚泊作业的定量风险评估 [J]. 天津理工大学学报, 2007, 23(5): 85-88. DOI: 10.3969/j.issn.1673-095X.2007.05.025. GUO Z B, ZHANG Q Z, XU H. Quantitative risk assessment of submarine pipeline in anchoring [J]. Journal of Tianjin University of Technology, 2007, 23(5): 85-88. DOI: 10.3969/j.issn.1673-095X.2007.05.025. [12] 王凤云, 赵冬岩, 王琮. 关于海底管线挖埋深度的规范标准研究 [C]// 中国海洋工程学会. 第十五届中国海洋（岸）工程学术讨论会, 太原, 2011. 北京: 海洋出版社, 2011: 407-410. WANG F Y, ZHAO D Y, WANG Z. Study on the standard of the depth of submarine pipeline excavation [C] //Chinese Ocean Engineering Society. The 15th Conference on China Ocean (Shore) Engineering, Taiyuan, 2011. Beijing: China Ocean Press, 2011: 407-410. [13] 马良, 王金英. 海底管道埋设技术论证 [J]. 中国海洋平台, 1999, 14(6): 17-20. MA L, WANG J Y. A demonstration on the laying and burying of subsea pipeline [J]. China Offshore Platform, 1999, 14(6): 17-20. [14] 马良, 王金英. 海底管道的埋设 [J]. 油气储运, 1993, 12(1): 27-30. MA L, WANG J Y. Embedment of subsea pipeline [J]. Oil & Gas Storage and Transportation, 1993, 12(1): 27-30. [15] 国家能源局. 城市电力电缆线路设计技术规定: DL/T 5221－2016 [S]. 北京: 中国电力出版社, 2005. National Energy Administration. Technical rule for design of urban power cables: DL/T 5221−2016 [S]. Beijing: China Electric Power Press, 2005. [16] 中山茂雄, 清宫理. 投锚与入土深度试验 [R]. 横须贺: 日本运输省港湾技术研究所, 1975. SHIGEO N, QING G L. Anchor dropping and soil depth test [R]. Yokosuka: Institute of Harbor Technology, Ministry of Transport, Japan, 1975. -
点击查看大图
图(4) / 表 (1)
计量
- 文章访问数: 175
- HTML全文浏览量: 14
- PDF下载量: 47
- 被引次数: 0
